There are 2 main
methods you can use to solve equations involving exponential and logarithmic
functions:
1) The “Key to Everything”
This is the definition
of a logarithm.
This property works
because exponential and logarithmic functions are one-to-one, meaning that for
every x value, there is only one
corresponding y value. In other
words, they pass the vertical line test.
To illustrate the one-to-one
property:
It is also helpful to
know the Inverse
Properties of logarithms:
We can show that this is true using the key to
everything.

Again, the key to everything helps us prove this. Rearranging the equation, we see that:

Knowing all the other
properties of logarithms, which can be found in the post on section 3.3, will also be very useful in solving
such equations.
Examples:
Solving Exponential Equations
1) Solve for x.
Given this
equation, we can simplify the right side:


Using the one-to-one property, we can then conclude that x=-5.
2) Solve for x.
Using the key to
everything, we find that:

Since a logarithm with a base of “e” is the same as a natural log, we simplify to:

The answer can
be written as x=ln3, which is
approximately equal to 1.099.
Solving Exponential Equations in Quadratic Form
Then, we factor
and solve like a regular quadratic:
Finally, using
the key to
everything, we find that:
*HOWEVER: The solution x=ln(-2) is extraneous,
because you cannot take the logarithm or the natural logarithm of a negative
number. Therefore, the only real solution to this equation is x=ln5.
Solving Logarithmic Equations
Using the one-to-one
property, we can simplify to:

Then, solve for
x as you would normally.

5) Solve
 .
.
We begin by
simplifying:
Then, using the key to
everything, we find that:
We can then
solve for x.













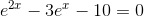










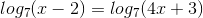

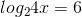

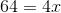
No comments:
Post a Comment