The goal of solving trigonometric equations is to isolate the trigonometric
function involved in the equation, this can be done using standard algebraic
techniques.
Solving a basic trig
equation
2sin(x)-1=0 Original equation
2sin(x)=1 Add one to each side
sin(x)=1/2 Divide each side by 2
Use the unit circle or sine
wave to find the solutions to this equation
According to the unit circle and sine wave
Therefore
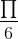
and
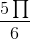
are
the
two solutions in the interval ⊏0,2∏).
If you want to express all possible solutions for this trig equation add 2n∏ (because the period is 2∏) to the end of each solution. For this
particular equation, there are infinitely many
solutions. (n is an integer)
Factoring Trig Equations
By taking out the cot(x), you can then use the zero product
property.
The solutions x=∏/2 and x=(3∏)/2 come from the equation
cot(x)=0
This equation needs to be simplified
further
Add 2 to both sides
and then take the square root of both sides.
*Watch out for equations that can have no solution because their solution is
outside the range of the function.
The real solutions to this equation is:
*Remember, the period of cot is ∏,
not 2∏. If you want to express all possible solutions
for this trig equation add n∏ to the end of each solution. For this particular
equation, there are infinitely many solutions. (n is an
integer)
*It would be redundant for this to
be the solution:(The first
equation is the simplified solution it is the only solution necessary for the
final answer.)
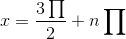
Sum and Difference of
Cubes
-Sometimes you will need to use the sum or difference formula in
order to solve trig equations. It is helpful to remember the phrase: Same,
opposite, always positive (S.O.A.P) when factoring.


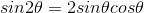






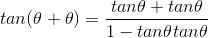




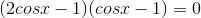

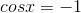

















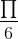
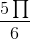







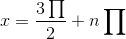



.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)
.gif)